The Trouble with the Turing Test
Notice: Undefined index: gated in /opt/bitnami/apps/wordpress/htdocs/wp-content/themes/thenewatlantis/template-parts/cards/25wide.php on line 27
On the fallacy of thinking computers

Alan Turing (1912–1954) was the mastermind of some of the most significant scientific discoveries and technological breakthroughs of the twentieth century. While he lived, the public had no idea that he was also a war hero whose astonishing feats of cerebration made him deserving of glory to rival that of the greatest Allied generals. Turing’s arrest and disgrace, on the other hand, were a public scandal, tabloid fodder. The fulfillment of his patriotic duty in which he distinguished himself was withheld from public knowledge, a state secret jealously guarded; his private life was laid bare in the press for all to gape at.
Today Turing is a hero several times over: the founding father of stored-program digital computing and artificial intelligence; the cryptanalyst who spearheaded the successful British effort to crack the Nazis’ unbreakable Enigma codes; the sufferer, perhaps, from borderline Asperger’s syndrome whose own quasi-autistic oddity he not only overcame but exploited as a strength; the martyr in the Oscar Wilde tradition whose persecution has inspired others to love without shame.
In such tours de force as the universal machine that bears his name — the computer that can do everything every other computer can do — and the imitation game — the test designed to show whether a machine can pass muster as a human intelligence — one sees the theoretical fundamentals of the practical wizardry that has made ours the age of unprecedented digital marvels. His professor, colleague, and friend M. H. A. Newman stated in a 1955 memoir of Turing for the annals of the Royal Society, “The central problem with which he started, and to which he constantly returned, is the extent and the limitations of mechanistic explanations of nature.” Newman is right to emphasize Turing’s lifelong exploration of an elemental abstract question; but it is important to note that, at full throttle, Turing joined theory and practice as few scientists have. The code-breaking machinery he devised during the war, and the programming system he invented after the war, were not mere satellites that revolved around the core theoretical concern of his scientific life; they were intellectual suns in their own right.
Turing’s gift for abstraction met his passion for tangible result in the work that excited him most. He was at home in the world that nature had made and that remarkable scientists like himself were reshaping, often to general mental consternation. As Turing mentally constructed his universal machine, the very foundations of mathematics — the basis for the modern understanding of the physical world — were called into question. As he pondered the similarities between the mind of man and the mind of the machine, the traditional meaning of our humanity was challenged.

Alan Turing was the second son of a mid-level administrator in the Indian Civil Service, and he was conceived in the port city of Chatrapur. In Alan Turing: The Enigma (1983), his brilliant if occasionally overzealous biographer Andrew Hodges writes with rather too evident portentousness that it was there “the first cells divided, broke their symmetry, and separated head from heart.” This skewing of thought and feeling would be a lifelong Turing hallmark, encouraged early on by the emotionally arid upbringing in the home of a retired Army officer and his wife, who raised Alan and his brother in England while their parents lived half a world away.
As befits a budding genius, the young boy’s mental life was precocious and passionate. It took him all of three weeks to teach himself to read from a primer. Numbers fascinated him even more than words. He would examine every lamp post he passed to note its serial number; what he did with those numbers once he possessed them is not known. At the age of ten he discovered that there was something called science, in a gift book called Natural Wonders Every Child Should Know by Edwin Tenney Brewster. The book introduced Turing to the mechanistic description of human life that would be his consuming theme: “For, of course, the body is a machine. It is a vastly complex machine, many, many times more complicated than any machine ever made by hands; but still after all a machine.” The human soul, which at that time was still spoken of in polite society, did not enter this elementary scientific picture; natural wonders had no place for such mystery, with its unacceptable flavor of the supernatural.
At Sherborne, a public (what Americans would call private) school of middling distinction, which Turing entered at thirteen, the authorities regarded science as wanting in the dignity of serious thinking, and as presumptuous in its confidence that it would explain the inexplicable. The headmaster, Nowell Smith, derided “the shallowest mind that can suppose that all the advance of discovery brings us appreciably nearer to the solution of the riddles of the universe which have haunted man from the beginning.” Andrew Hodges for his part derides “the miniature, fossilised Britain” represented by this blinkered contempt for the vanguard intelligence of modernity. It is true enough that for a mind such as Turing’s the atmosphere was stiflingly uncongenial. The headmaster laid down the law in a report to his parents: “If he is to stay at a Public School, he must aim at becoming educated. If he is to be solely a Scientific Specialist, he is wasting his time at a Public School.” Whether this vaunted education meant something more serious at Sherborne than drilling Latin grammar and fortifying the imperial character is unclear. In any case, young Turing showed himself serious after the manner of a mathematician and scientist born and bred. To question unrelentingly the accepted truths — to follow the example of Einstein, whom Turing revered for daring to doubt the axioms on which the Newtonian structure of the known world was founded — came naturally to his temperament.
For all his mental boldness and address, however, he would display the characteristic limitations of a scientific specialist. To devise a universal machine, one needn’t be a universal man after the Leonardo or Goethe model; but to recognize the profound mystery that remains after one has examined the similarity between the human mind and a man-made machine, it helps to be educated beyond the reach of scientific specialization. Turing was certainly a genius, but he was not a many-sided one. Renaissance men were not thick on the ground even during the Renaissance, and perhaps after Goethe such masterly versatility was no longer possible. With the exponential growth of scientific knowledge since his time, anyone aspiring to embody every intellectual excellence is necessarily doomed to dilettantism, and in the strictly pejorative sense of that word. Turing’s mind played to its strengths, and it was superbly competent within its orbit. But it was not without its limits.
At fourteen Turing found that his mind and heart could be thrilled in perfect unison. Christopher Morcom, a small, fragile-looking, and exceedingly clever boy a year ahead of him at school, was his first love, and his only love truly deserving the name. A shared passion for mathematics provided Turing the avenue of approach. As it happened, Christopher was a far more successful student than Turing in math, and everything else. Turing wrote that he “worshipped the ground [Christopher] trod on.” Hodges describes this love of Turing’s as “the first of many for others of his own sex,” but in fact none of Turing’s biographers shows him in love ever again in his life; convenient affairs and abrupt couplings were the rule. His necessarily chaste love for Christopher, who apparently was not gay, stood apart where matters of the heart were concerned. In the richly suggestive novel A Madman Dreams of Turing Machines (2006), Janna Levin gets right the momentous uniqueness of Turing’s longing for Christopher: “Chris is Alan’s first, greatest, purest, entirely unrequited love.”
Christopher’s sterling moral and intellectual example made Turing undertake to improve his own schoolwork and character. He hoped to join Christopher at Trinity College, Cambridge. As Hodges notes, “In mathematics and science, Trinity held the highest reputation among the colleges of the university which was itself, after Göttingen in Germany, the scientific center of the world.” Along with his friend, Turing put in for a prestigious and lucrative scholarship at Trinity, though he was a year younger and less well prepared than the usual candidate. Together at Cambridge for the entrance examinations in December 1929, the two youths enjoyed what Turing called the happiest week of his life. But Christopher was awarded a Trinity scholarship, and Turing fell short; he would have to wait another year and try again. The prospect of a year without Christopher stretched before him with Saharan bleakness.
And then Christopher died, in February 1930. His obvious frailty had been symptomatic of bovine tuberculosis, caught from tainted cow’s milk when he was a young boy. Turing had had no idea his beloved was so ill, and he was desolated. To Christopher’s mother he wrote, “I am so glad the stars were shining on Saturday morning, to pay their tribute as it were to Chris. Mr O’Hanlon had told me when [the funeral] was to take place so that I was able to follow him with my thoughts.” To his own mother he expressed the abiding hope that this friendship was to be graced with eternity, and he vowed to make himself worthy of Christopher’s companionship in the world after this one: “I feel sure that I shall meet Morcom again somewhere and that there will be some work for us to do together, and as I believed there was for us to do here. Now that I am left to do it alone I must not let him down but put as much energy into it, if not as much interest, as if he were still here. If I succeed I shall be more fit to enjoy his company than I am now.” For young Turing, true love meant everlasting life, and ceaseless Faustian striving, for spirits meant to be conjoined. His soul yearned for permanence and perfection, and at least for a time his mind followed obediently.
The next December Turing applied again for a Trinity scholarship, and once again he failed; but he was named a scholar at his second-choice Cambridge college, King’s, where he would study pure and applied mathematics. Applied mathematics meant the basis of theoretical physics; Cambridge ranked just below Göttingen in innovative work on the quantum world of subatomic particles. In the British tradition of undergraduate specialization, Turing was no longer expected to become educated across a range of disciplines, and yet he would continue his metaphysical speculations about the intimate relations of body and spirit.
He did his utmost to reconcile his eternal longings with the latest scientific discoveries. As he wrote in a letter to Mrs. Morcom, the nineteenth-century physicist’s ideal (famously described by Laplace), in which knowing the position and momentum of every object in the universe would enable the panoptic mind precisely to plot the future to the last detail, had been displaced by indeterminacy on the scale of the infinitesimal. And for Turing, subatomic indeterminacy also suggested freedom for human beings.
We have a will which is able to determine the action of the atoms probably in a small portion of the brain, or possibly all over it. The rest of the body acts so as to amplify this. There is now the question which must be answered as to how the action of the other atoms of the universe are regulated. Probably by the same law and simply by the remote effects of spirit but since they have no amplifying apparatus they seem to be regulated by pure chance. The apparent non-predestination of physics is almost a combination of chances.
From the freedom of the human will and the anarchic concatenations of the quantum world, Turing leaped to reflection on the unity of body and spirit. He had moved on from his faith in disembodied spirit to confidence that spirit detached by death from one body will find another to inhabit: “Personally I think that spirit is really eternally connected with matter but certainly not always by the same kind of body. I did believe it possible for a spirit at death to go to a universe entirely separate from our own, but I now consider that matter and spirit are so connected that this would be a contradiction in terms. It is possible however but unlikely that such universes may exist.” The loss that continued to gnaw at his vitals directed Turing into meditations more religious than scientific. He took comfort wherever he could find it, as those bereft have always done.
He also took pleasure in the various activities Cambridge offered to a young man eager to try every diversion. In Turing: Pioneer of the Information Age (2012), B. Jack Copeland rattles off the numerous ways Turing kept himself entertained: “Young Turing rowed, played bridge and tennis, networked, enjoyed the theatre and opera, played his second-hand violin. He skied, slept under canvas, dreamed of buying a small sailing boat, travelled in Europe, joined the Anti-War Movement.” The networking included the expansion of sexual possibilities. In 1885 same-sex relations were tarred as the criminal offense of “gross indecency,” violators were subject to imprisonment, and the law remained on the books until 1967. As a character in E .M. Forster’s novel Maurice remarks of this social disapprobation, “England has always been disinclined to accept human nature.” In an England largely hostile to gay people, Cambridge provided them a haven of sorts: the Bloomsbury Group, which became known for celebrating same-sex relationships, and included as members Forster and the economist John Maynard Keynes.
In The Man Who Knew Too Much: Alan Turing and the Invention of the Computer (2006), David Leavitt writes that Turing was remarkable even by Cambridge standards for his willingness to declare himself exactly who he was: “Even within the protective walls of King’s, to be as open about one’s homosexuality as Turing was either insane or revolutionary. Or perhaps it was simply logical — further evidence of his literal-mindedness, his obliviousness to the vagaries of ‘the world.’ Turing neither glorified nor pathologized his own homosexuality. He simply accepted it and assumed (wrongly) that others would as well.” Turing was able to take a disinterested view of his sexual nature, and he described as simply as possible what he saw, without grasping for reasons why things should be as they are or wishing they could be any different. If nature made him the way he was, it would be an intellectual error to call his sexuality unnatural. That respectable people might think otherwise did not diminish his self-respect.
The integrity of his understanding mattered immensely to the emerging mathematician. Even as an undergraduate Turing was known for remaining oblivious of the published literature and figuring out his own method of conceiving proofs. After faltering academically in the early going, he demonstrated his supreme competence in his final exams in 1934, being designated a B-star Wrangler — Cantabrigian parlance for a hotshot. In 1935 he presented a dissertation, “On the Gaussian Error Function.” In it he included his proof of the central limit theorem, which explained the way measurements fall into place to produce the statistical bell curve. Although someone else had already proved the theorem more than a decade before, Turing’s version was sufficiently novel and elegant to impress the authorities, and King’s named him a Fellow at the startling age of twenty-two. Admirers celebrated his achievement with an ambiguous morsel of verse: “Turing / Must have been alluring / To get made a don / So early on.”
Biographer Andrew Hodges, an Oxford mathematics don, describes in masterly fashion the mental whirl that Turing confronted as he explored the logical foundations of his chosen subject. Hodges writes that mathematics had once been presumed to represent material facts. But the discovery, or invention, of such counterintuitive concepts as negative and imaginary numbers had flummoxed that basic understanding, and by the nineteenth century inaugurated the tendency “in many branches of mathematics towards an abstract point of view. Mathematical symbols became less and less obliged to correspond directly with physical entities.” Once this disconnection of symbol from material object became the vogue, “symbols might be used according to any rules whatever,” and nihilism infect the purest branch of human thought. “If [mathematics] was to be thought of as a game, following arbitrary rules to govern the play of symbols, what had happened to the sense of absolute truth?”
The Cambridge eminence Bertrand Russell examined the plight of absolute truth and the perilous incoherence of the game of symbols in his Introduction to Mathematical Philosophy, which came into Turing’s hands in 1933. In the accepted axioms of set theory, which had become foundational for the rest of mathematics, Russell had discovered a contradiction. The axioms were modified to eliminate that inconsistency, but it was not unreasonable to fear that others might be lurking. A mathematical system based on logic demands consistency, as Hodges explains: “If one could ever arrive at ‘2+2=5’ then it would follow that ‘4=5,’ and ‘0=1,’ so that any number was equal to 0, and so that every proposition whatever was equivalent to ‘0=0’ and therefore true. Mathematics, regarded in this game-like way, had to be totally consistent or it was nothing.”*
No mathematician or scientist can abide a line of thought that amounts to nothing, to nonsense. The most emphatic assertion of the need for strict regulation of the formalist mathematical game came from David Hilbert, Göttingen’s brightest star, considered by some in his day the most influential geometer since Euclid. What became known as “Hilbert’s program” was the project of creating a system that satisfied three goals. Completeness demanded that every statement could be proved or disproved. Consistency required that no proof could lead to a contradiction. Decidability mandated that a procedure existed to determine whether or not any given statement was provable.
Hilbert’s program for mathematical certainty bore a stern but heartening moral imperative. “Wir müssen wissen, wir werden wissen,” was his hortatory byword: We must know, we shall know. And when it comes to mathematics and natural science, Hilbert asserted, “there is no such thing as an unsolvable problem.”
Hilbert’s prospectus for the glorious future would not be realized. In 1931, the Austrian logician Kurt Gödel controverted two of Hilbert’s three crucial postulates, completeness and consistency; decidability remained an open question, but not for long. The concept of a systematic procedure required for decidability is typified by multiplication or long division — in biographer B. Jack Copeland’s words, “simply a paper-and-pencil method that anyone can carry out, step by mechanical step, without the need for any creativity or insight.” Such procedures were routinely performed in countless offices by clerks doing calculations; these human drones were known as computers. The systematic procedures they followed were mechanical in a precise sense: they could be done by machines.
In 1935, while Turing awaited the examiners’ judgment on his dissertation, he attended a lecture series on Hilbert’s program. After the lecture, Turing went off on his own, as was his custom when his mind was alight, to think over the ramifications. His ponderings launched him on a line of inquiry that would eventually lead, in the hands of others, to the computer age.
A year later, he produced the astonishing paper “On Computable Numbers, with an Application to the Entscheidungsproblem” — the decision problem that Hilbert had posed. Hilbert imagined that there existed a supreme systematic procedure that would prove every mathematical statement in a system true or false, and thereby establish mathematics at last “on a concrete foundation on which everyone can agree.” In 1928, one learns from Copeland in The Essential Turing, Hilbert identified the decision problem as “the main problem of mathematical logic” — very difficult, as yet unsolved, and its solution a matter of “fundamental importance.” Here was the number-crunching version of The Key to All Mythologies, or, as M. H. A. Newman called it, a “new philosopher’s stone.” Turing would prove Hilbert’s master idea a chimera, and complete the demolition of his prospective system that Gödel had begun.
With an ironic fillip, Turing proved the impossibility of “a completely mechanized mathematics,” in Copeland’s phrase, by inventing a concept for a universal machine. Turing begins his classic 1936 paper, “The ‘computable’ numbers may be described briefly as the real numbers whose expressions as a decimal are calculable by finite means…. According to my definition, a number is computable if its decimal can be written down by a machine.” The machine can be said to resemble someone performing a rote calculation. Just as the human computer works with paper and pencil, the machine has a tape analogous to paper running through it, but limitless in length. Turing calls a machine whose configurations completely determine its every move an automatic machine; once it starts, its actions are not subject to human modification. It can perform computations of fiendish complexity.
All of its configurations and operations would be written out in a table of behavior, which contains all the necessary information for the machine to work, whether it is actually built or not. “From this abstract point of view, the table was the machine,” Andrew Hodges writes. “There would be infinitely many possible tables, corresponding to infinitely many possible machines,” now called Turing machines, though their maker was not so full of himself as to name them that. And there was the marvelous factotum that can do anything any other machine can do, a universal Turing machine.
Turing would ask whether this machine that can perform all possible computations “could produce the decision that Hilbert asked for,” in Hodges’s words. In essence, Turing solved the decision problem by showing that there is no systematic procedure for determining whether any given Turing machine will eventually halt or will instead run forever. The exhaustive brute force attack of which the machine was capable would not have force enough to decide the matter in a finite time. It is only a technical matter to translate that result into a demonstration that there is no way to decide whether a given mathematical statement is provable or not. Hodges concludes: “Alan Turing had dealt the death-blow to the Hilbert programme. He had shown that mathematics could never be exhausted by any finite set of procedures.”
M. H. A. Newman was agog at his student’s theorization of a machine, of all things, to solve the most abstract of questions: “It is difficult to-day to realize how bold an innovation it was to introduce talk about paper tapes and patterns punched in them, into discussions of the foundations of mathematics,” he wrote in Turing’s memorial address. Cambridge mathematicians did not expend their mental powers on anything so vulgar as machinery. At Cambridge, even applied mathematics pertained to the most abstruse theoretical physics; one might consider the electron in certain of its arcane manifestations, but would not easily turn one’s thoughts toward its application in so-called electronics. Engineering, invention, were the purview of a lesser breed, who did not mind the perpetual grime around their fingernails. The progeny of polytechnic institutes and trade schools concerned themselves with such matters. Mathematizing gentlemen forbore.
Turing’s breakthrough paper would make him a lasting name, eventually; but for the moment he was upstaged by the American logician Alonzo Church, whose take-down of Hilbert’s decision question was published just before Turing’s. Once again, Turing had been beaten to the punch, but once again his work was impressively unique despite the points of similarity. It was published and in due course not only became the definitive word on the subject, but, with the idea of his machine, also conceived an entirely new field. In a review essay, Church named the universal machine in Turing’s honor.
Turing was shedding his old skin. The material world, which he had regarded as principally the home of eternal spirit, was acquiring an overwhelming reality, and crushing the life out of his religious fancies. The love that survived death had made him confident that the spirit is everlasting, but as his thoughts began to cool he thrashed his way toward a strictly logical point of view. If his machine could be said to have “states of mind,” as he had written, perhaps the analogy could profitably be extended: As the machine worked on the model of the human intelligence, doing what the bureaucratic human computer can do, then the question of how far the human mind could be called a mechanism seemed a field of inquiry charged with promise. As Hodges writes, “Turing would soon emerge as a forceful exponent of the materialist view and identify himself as an atheist. Christopher Morcom had died a second death, and ‘Computable Numbers’ marked his passing.”
Janna Levin, in her deeply moving and perceptive novel about the parallel lives of Turing and the Austrian logician Kurt Gödel (who put the first nail in the coffin of Hilbert’s mechanized mathematics), imagines Turing violently purging his mind of the intolerable residue of spiritual yearning that had outlived its intellectual and emotional value:
He finds an infinite list of mathematical facts that can never be proven. Uncomputable numbers forever beyond human reason.
…. His materialism escalates with these incredible epiphanies even as his awkward faith cloys and whines and nags him into misery. His materialism versus his faith. With a kind of morbid fascination, Alan stares at the brutal flaying of his beliefs with pity and a smudge of contempt for the loser….
The human mind can also be reduced to a machine. This idea drives all the others as he runs on grass, past trees, over bridges, through cattle. States of the mind can be replaced by states of the machine. Human thought can be broken down into simple rules, instructions that a machine can follow. Thought can be mechanized. The connection isn’t perfectly clear, but it is there, the catalyst of a great crystal. It is not just that thought can be mechanized. It is mechanized. The brain is a machine. A biological machine….
He runs along the Cam, trampling familiar spots where he mourned Chris. His stride is smooth and easy and confident when the admission forms completely: Where is God in 1+1=2? Nowhere.
…. We’re just machines.
Levin’s psychological insight seems that of a born novelist and runs rather contrary to the ethos of the scientist she also is: Turing’s conversion to materialism and atheism, which have become standard issue for scientists, relieves him of an emotional encumbrance, permits him to live more freely in both mind and body, undisturbed by immortal longings for impossible loves. Psychic comfort seems to matter to him at least as much as truth. His is the characteristic liberating disillusionment of a certain type of modern intellectual, who casts off clamoring spiritual need and chooses to live for a purely mental discipline that also happens to license the body’s unconstrained satisfaction. Levin’s Turing is renouncing an exquisite loving sorrow and a profound search for God in order to embrace the mind’s exalted austerity and the body’s indiscriminate importunity. One might say in his defense that he is choosing ordinary, reasonable, fully modern life over archaic death-haunted convention; but it does not appear altogether a winning bargain. Hodges, Leavitt, and Copeland on the other hand all seem to think that this choice represents a step unmistakably in the right direction, healthy growth toward mature understanding and even wisdom. They do not waste energy mourning what is lost in the transaction.
In September 1936 Turing sailed off to the United States and would spend two years at Princeton. Princeton’s status had risen, as that of Göttingen and other German universities declined with the flight toward freedom of eminent professors, many of them Jewish. As Turing wrote to his mother, between the mathematics department and the Institute for Advanced Study, Princeton had collected “a great number of the most distinguished mathematicians here. J. v. Neumann, Weyl, Courant, Hardy, Einstein, Lefschetz, as well as hosts of smaller fry.” Church was the foremost logician there, and Turing faithfully took in his lectures; but to Turing’s regret, Kurt Gödel had left the year before, and the two of them would never meet.
Turing wrote a Princeton doctoral dissertation, “investigating whether there was any way of escaping the force of Gödel’s theorem,” in Hodges’s words. “The fundamental idea was to add further axioms to the system, in such a way that the ‘true but unprovable’ statements could be proved.” He resorted to discussing the addition of an infinite number of axioms, and did not resolve the problem to his own satisfaction. But his examiners, including Church, noted his overall excellence in awarding him the degree.
Even in tranquil Princeton the threat of barbarism impinged upon minds of great refinement and sublime unworldliness. Hodges foreshadows the storm that would soon break upon the world, quoting from one of Turing’s letters home in which he addressed the question “What is the most general kind of code or cipher possible,” and boasted a little about the codes he had devised. One code in particular was “pretty well impossible to decode without the key, and very quick to encode,” and this one with some others might earn him a tidy sum if he were to sell them to His Majesty’s Government. But he was “rather doubtful about the morality of such things. What do you think?” The peaceably-minded British mathematician G. H. Hardy, in his 1940 essay “A Mathematician’s Apology,” added his own touch of dramatic irony to Hodges’s discussion of the practical uses of mathematics: “No one has yet discovered any warlike purpose to be served by the theory of numbers or relativity, and it seems very unlikely that anyone will do so for many years.” The moral questions and qualms would be set aside by and by as the military usefulness of higher mathematics became evident.
The Government Code and Cypher School (GC&CS), which operated under the aegis of the Foreign Office and its espionage arm MI6, also known as the Secret Intelligence Service, had its beginnings in the Great War. The outfit had then been known as Room 40, and was affiliated with the Admiralty, which was headed by the First Lord thereof, Winston Churchill, already a zealous advocate for the importance of cryptography. The School’s educational project was straightforward but discreetly veiled in official euphemism; with the euphemism removed, its mission was to crack the codes of the enemy, and no doubt of some allies in the bargain, and to safeguard Britain’s own codes against foreign intrusion. Spycraft in this department was largely a gentleman’s pursuit. Before the Second World War, cryptanalysis had been the province of Oxbridge-trained classical scholars, some of them experienced in and adept at unknotting the linguistic tangles on ancient papyri. Mathematicians had been thought simply wrong for this work — they were flighty, disorganized, unreliable — and as late as 1938 not one was employed by the GC&CS.
That would change drastically, and all for the better. When Turing returned to England in the summer of 1938, he was tapped to attend a course at the GC&CS headquarters in London — how he was recruited is unclear, but they got the right man for the job.
Also in 1938 the estate known as Bletchley Park was bought by the government with an eye toward eventualities: GC&CS could not prudently be left in London, which was rightly believed to be the future cynosure of Nazi air attack. Bletchley Park, halfway between Birmingham and London, and between Oxford and Cambridge, would become legendary, but only long after the fact, because the strictest secrecy was enjoined upon those who worked there. When recruitment began in earnest, GC&CS had about 30 codebreakers and 150 intelligence and support staff. “It was swiftly understood in 1938 that rather more were going to be needed,” the journalist Sinclair McKay writes with deft understatement in The Secret Lives of Codebreakers: The Men and Women Who Cracked the Enigma Code at Bletchley Park (2010). By war’s end the number of workers had reached some ten thousand, and the cryptanalysts there, Turing most prominent among them, had penetrated layer upon layer of cryptographic armor plate.
The German cryptographic system known as Enigma was patented by the electrical engineer Arthur Scherbius in 1918, and soon introduced into commercial service, used by several banks to protect their secret communications. In 1926 the German navy adopted Enigma, and fortified the machine for warlike purpose; the German air force and then the army proceeded to acquire it as well. Removed from the open market, it became the exclusive property of the German military, which modified the system still further, and took pride in its apparent invulnerability. Each branch of the armed forces had its own variation of the machine; the navy’s version was the most formidably complex.
Sinclair McKay describes the machines as “compact, beautifully designed devices, looking a little like typewriters with lights.” B. Jack Copeland praises the machines’ adaptability to a variety of wartime uses, as an advertiser might pitch the multiform practicality of an especially clever gizmo useful in your house, garden, and automobile: “Lightweight and highly portable, the Enigma machine was equally at home in a general’s office in Berlin, an armoured vehicle, a submarine, or trench.” The apparatus comprised a typewriter-style keyboard displaying the twenty-six letters of the alphabet; a lampboard or “lightboard” displaying the alphabet’s letters as lights rather than pressable keys; a plugboard, resembling an old-school telephone switchboard, on which the letters could be connected through wires; and removable rotating wheels, each of which had the alphabet printed on it in a ring along the rim.

The rotating wheels (typically three of them) were all wired differently, and those that were to be used on a particular day were selected from a larger collection. The settings list provided in a printed booklet, new each month, instructed all the Enigma operators in a particular network as to the prescribed wheel order and plugboard connections, whose positions changed each day at midnight. Hugh Sebag-Montefiore provides an account of the system’s workings in Enigma: The Battle for the Code (2000):
An Enigma was used to scramble the letters making up the words in a message before it was sent out in Morse code by a radio transmitter. So, for example, if a German wanted to send a message saying “Hitler ist in Wilhelmshaven,” the Enigma operator would tap the H key on his keyboard and write down on his notepad which bulb on the Enigma’s lightboard lit up. And so on for each letter of the message…. Hitting a key on the Enigma keyboard released an electric current which ran to a series of scrambling elements — including a plugboard and three wheels. The scrambling elements diverted the current away from its original course. The current would then hit a “reflector” end disk which would send the current back through the same scrambling elements again, though on a different course, and the current would finish up by lighting a bulb marked with one of the letters of the alphabet.
The Enigma operator would typically team up with a radio operator who would send the occult ciphertext. The operators receiving the message would reverse the procedure. The radio operator would translate the message from Morse code into the alphabet salad of ciphertext, and the Enigma operator, who had adjusted the machine to the settings specified for the network in the orders of the day, would type in the ciphertext at the keyboard; the original German text would then appear one letter at a time on the lightboard.
The conception sounds relatively simple, but the execution was dauntingly tortuous and confounding to any Allied cryptanalyst trying to unscramble the message. The wheels alone, in their most common configuration, presented the would-be infiltrator with 105,456 possible settings. The plugboard connections multiplied the possible states of the machine to the point of cerebral explosion on the interloper’s end: According to Hodges, there were 1.3 trillion possible plugboard connections for each of the 105,456 settings of the wheels. And as Copeland notes, “this presupposes that the codebreaker had already found out, somehow, the basic details of the machine — in particular what the wiring arrangements were inside the wheels.” The Enigma machine was a triumph of German engineering. It looked to be an unbeatable weapon.
By 1932, French and British intelligence officers had obtained, through the arts of spycraft and skullduggery, operating manuals and settings lists for the Enigma; yet their cryptanalysts were unable to break the cipher, so they entrusted the purloined materials to Polish experts. One of them, Marian Rejewski, used the trove to puzzle out the Enigma wiring, and the Poles capitalized on this breakthrough to read the German transmissions. For six years, however, they failed to inform the French or the British of their discoveries. Only when war was imminent and Poland was in the Nazi crosshairs — and after the German navy changed its Enigma system and the Poles lost access to that line of traffic — did they divulge their findings to their friends. While Bletchley Park was reading German armyand air forcemessages within twenty-four hours of interception by 1940, the navy’s Enigma would remain a closed book to the British until 1941.
Turing began to open that closed book just a crack in 1939, and thereby opened vistas of possibility whose realization would change the course of the war. He was one of very few British cryptanalysts working on Enigma already months before the war began. And he was operating on his own, as he preferred to do; collegial input he found more hindrance than help. For the so-called Dolphin network of the Naval Enigma, which the U-boats used, Turing managed to deduce “the tricky method used to tell the receiving operator what positions the sender’s wheels were in when he started enciphering the message,” Copeland writes. The Dolphin network, unlike others that were simpler, used two stages of encryption to tell the receiver the position of the sender’s wheels when the message was encrypted. One encryption used the Enigma machine. The other was done by hand with instructions shared by all operators, using a chart that specified how any given pair of letters should be encoded — known as a “bigram table.” The receiver then needed to use first the bigram table and then the Enigma machine to reverse-engineer the position of the sender’s wheels and decrypt the message.
Beginning with several messages that the Poles had succeeded in decrypting two years before, Turing figured out how the process workedone night in late 1939. The significance of what he found, Copeland asserts, can hardly be overstated. “The night of Turing’s break, whose exact date was unrecorded and whose discoveries remained shrouded in secrecy for nearly sixty years, was undoubtedly one of the most important nights of the war.” Nevertheless, he still lacked the information that would make U-boat messages transparent: He had to get the network’s bigram tables. Any other information he could get would make the assignment that much easier. That procurement would be the job of men on the high seas.
“The only thing that ever really frightened me during the war was the U-boat peril,” Winston Churchill would write in his most famous military history. The decisive theater of war, in his opinion, was the North Atlantic. The Nazi submarine campaign against British merchant ships, which provided the lifeline from the United States and Canada, and which traveled in convoys under the protection of warships, had become so effective by the autumn of 1940 that Britain was in danger of being “starved into submission,” as Sebag-Montefiore puts it. Breaking Enigma would reveal the positions and intentions of U-boats, which took to hunting in so-called wolf packs, and would thus enable the convoys to evade them.
The codebreaking could not have been accomplished by mathematical genius alone — not Turing’s, not even the whole Bletchley crew’s. The indispensable information on which the cryptanalysts worked their marvels came from successive small victories on the ocean. Brave sailors seized the German machines and documents without which the brainiacs would have been at a loss.
Shrewd guesswork, they believed, would play a critical part in deciphering Enigma. The so-called crib was a port of entry to the system — “a snatch of plain German that the codebreaker thinks could form part of the message,” in Copeland’s words. “The weather stations regularly sent messages containing routine phrases like ‘weather for the night’ … and ‘situation eastern Channel.’” “Armed with a good crib and vast patience, it was possible to fiddle with an Enigma machine until the message decoded — a procedure known simply as ‘twiddling.’” However, good cribs were hard to come by, and twiddling was not exactly an efficient technique. In November 1939, Turing and three associates signed a note to a higher authority describing their predicament. “Without cribs, they could not break any Enigma messages. If they could not break some Enigma messages, they would not be able to identify any cribs. The only other way to read Naval Enigma, as was acknowledged by Turing and his colleagues, was if settings were ‘captured from a submarine.’”
So it was not the cribs that worked in the early going; what Turing needed to jump-start the process was a pinch — the seizure of a German vessel with its Enigma machine and settings list and bigram tables. These too were hard to come by. The lack of progress maddened some of Turing’s colleagues, who blamed the failure on his sheer incompetence. Frank Birch, head of the section focused on the interpretation of Naval Enigma intelligence, and a historian by training, was howling loudly by August 1940 about the uselessness of mathematicians: “Turing and [his assistant Peter] Twinn are brilliant, but like many brilliant people, they are not practical. They are untidy, they lose things, they can’t copy out right, and they dither between theory and cribbing. Nor have they the determination of practical men.”
But Turing would prove heroically practical and determined. In due course the British Navy would provide him with the desired materials from captured submarines and other warships; whenever the German navy altered its Enigma settings, as it did several times during the war, the Brits managed to acquire the renewed necessities. Sebag-Montefiore details these daring seizures in his book, and the reader cannot but relish the tales of noble fearlessness and share the author’s excitement. Turing would exploit the captured Enigma materials, and the cribs that their possession made possible, in devising the electromechanical computers called bombes in early 1940. “At superhuman speed, a bombe searched through different possible configurations of the Enigma’s wheels, looking for a pattern of keyboard-to-lampboard connections that would turn the coded letters into plain German,” as Copeland writes. Marian Rejewski had developed a sort of prototype of Turing’s bombe that went by the name bomba in Polish, but his machine was primitive by comparison, and no longer worked once the Germans introduced two more possible wheels into the system.
Turing’s great innovation, which circumvented this problem, was to rely on a cunningly selected crib to initiate the action. Thirty Enigma replicas were joined together, in a metal cabinet over six feet tall and seven feet long, using ten miles of wire and over a million soldered joints. Hodges remarks that Turing’s “serious interest in mathematical machines, his fascination with the idea of working like a machine, was extraordinarily relevant.” With his “insight into the problems of embodying logical manipulations in this kind of machinery,” Turing was singularly qualified to head this operation. Geared for a specialized purpose in the original cyberwar and reliant on particular cribs, the bombe was not a Universal Turing Machine, but it impressively represented his mathematical and mechanical genius.
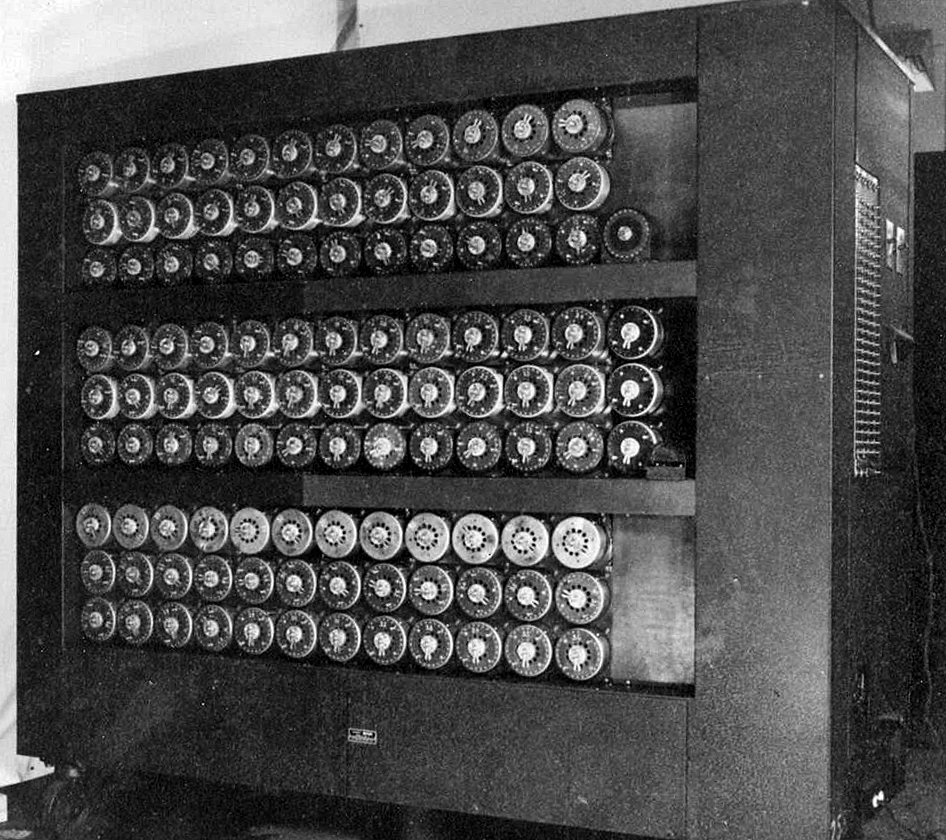
Although the first bombe was constructed at Bletchley Park in early 1940, its usefulness was severely limited at first, and only a year later would Enigma decryption and intelligence analysis, codenamed Ultra on the British end, issue in a major victory. In September, codebreakers cracked Italian naval Enigma, which did not use a plugboard and was accordingly less diabolical than the German naval system. In March 1941 intelligence analysts drew the connection between an Italian naval transmission and a Luftwaffe message, and notified the Admiralty of their discovery. Admiral Sir Andrew Cunningham, Commander-in-Chief of the Mediterranean Fleet, was given “enough evidence with which to make an educated guess,” as Sebag-Montefiore puts it, about the Italian fleet’s planned movements, and he set up an ambush. At the Battle of Matapan, Cunningham would write, “Five ships of the enemy fleet were sunk, burned or destroyed…. Except for the loss of one aircraft in action, our fleet suffered no damage or casualties.”
Thanks to subsequent Enigma breaks, the enemy would get repeated rough handling — to the point that the Germans had to suspect their codes had been compromised. Yet confidence in their invincible engineering led them again and again to overlook these suspicions — they were inclined to blame an utterly imaginary spy network in occupied France — and thus to pursue their own ruin. The British took full advantage of the enemy’s willful ignorance. By 1942, Hodges writes,
Bletchley Park was no longer outside the ordinary channels: it dominated them. Its productions were not the spice added to some other body of knowledge. It was nearly all that they had — photo-reconnaissance and POW interrogation adding points of important detail but never matching in scale what they had fresh from the horse’s mouth. There were sixty key-systems broken, producing fifty thousand decrypted messages a month — one every minute…. The soaring imagination of the analysts, exhausting the colours of the rainbow, had plundered the vegetable and animal kingdoms [to name the broken keys]: Quince for the SS key, Chaffinch for Rommel’s reports to Berlin, Vulture for the Wehrmacht on the Russian front.
And by D-Day, June 6, 1944, naval Enigma, the most difficult system to crack, “is being read almost currently,” Sebag-Montefiore declares. “This enables Bletchley Park to make sure no wolf pack attacks are being planned by U-boats, and it also means that Churchill and the Allied commanders can be warned as soon as the invading forces are spotted by the Germans.”
The work Turing did at Bletchley Park was eminently well-suited to his soaring intellectual gifts in concert with the earthly side of his temperament. Hodges, Leavitt, and Copeland all remark his need to conjoin theoretical discovery with practical application or invention. His intellect naturally ran to the most ethereal and icy reaches of mathematical abstraction, and he was at home in the upper limits of the intellectual stratosphere where thought sears at absolute zero and ordinary minds don’t stand a chance of lasting; but he wanted to be able to touch what his high-flying mind could see. The task to which the war directed his powers exercised both these aspects of his nature. “Indeed,” writes David Leavitt, “as each bombe clicked its way through thousands of eliminations and checks each day, it was as if the heartbeat of logic itself was being heard.”
It was not simply the love of knowledge for its own sake that drove Turing now in his passionate exertions, but the terrible urgency of life and death for millions in the balance. While Turing’s mental striving had been intense in the years before the war, now it was all-consuming. He was not out to answer eternal questions at Bletchley Park; the math he was doing there did not rival the recondite purity and consummate audacity of the answer to the “decision problem” that had earlier preoccupied him.He was engaged in a mental contest with a formidable enemy who posed him a practical problem, and at stake was the survival of civilization.
Indeed, General Eisenhower, the Supreme Allied Commander in Europe, declared after the war that the achievement of Bletchley had shortened it by two years. F. H. Hinsley, an expert Bletchley hand, estimated three. Some fifty million people were killed during the six years of the war, so the lives saved were a multitude of heroic proportion. And one of Turing’s most distinguished colleagues stated that without him the Allies might not have won the war at all.
The demands of war concentrated and clarified fundamental ideas of Turing’s that he had been deeply immersed in during his peacetime intellectual life. During his last term at Cambridge in 1939, Turing had not only taught a course on Foundations of Mathematics but had also attended a course with the same title taught by the philosopher Ludwig Wittgenstein. The courses were alike in name alone. Wittgenstein was conducting a frontal assault against mathematics as Turing understood it: “I shall try again and again,” the Viennese master lectured, “to show that what is called a mathematical discovery had much better be called a mathematical invention.” His biographer Ray Monk writes in Ludwig Wittgenstein: The Duty of Genius (1999), “There was, on his view, nothing for the mathematician to discover. A proof in mathematics does not establish the truth of a conclusion; it fixes, rather, the meaning of certain signs…. Wittgenstein presumably thought that if he could persuade Turing to see mathematics in this light, he could persuade anybody.”
In the end neither man could persuade the other. Wittgenstein frequently aimed his observations directly at Turing, as though he were the only auditor deserving his attention. The sort of paradox that Turing had relied on in his startling decidability proof, Wittgenstein argued, was of no practical consequence. “Because the thing works like this: if a man says ‘I am lying’ we say that it follows that he is not lying, from which it follows that he is lying and so on. Well, so what? You can go on like that until you are black in the face. Why not? It doesn’t matter.” To Turing it mattered a great deal: The contradictions in a mathematical system may well become lethally significant, he shot back, if “there is an application, in which case a bridge may fall down or something of the sort.”
Mathematical truth was a matter too important to be left to the philosophers. One might even say it was too important to be left to the pure mathematicians. The most abstruse and elegant theorem mattered because it bore directly upon brute fact, inelegant material reality. The mathematical mind can see what it sees on the heights, Turing believed, just as the ordinary man can touch everyday objects and know exactly what they are, and how many of them there are. These are the natural modes of human knowing, and one implicates the other. The world of esoteric abstraction available to a chosen few is no less real, but also no more real, than the world familiar to every man, in which he can be assured of his own reality and that of everything around him. And nothing else brought this manifold truth home to Turing as tellingly as did the consequence of his wartime duty.
The novelist Neal Stephenson in Cryptonomicon (1999) describes Turing at Bletchley as the perfectly sound-minded and preternaturally perceptive intellectual, who integrates the virtual otherworldliness of abstraction with earthbound solidity:
If he would just work with pure ideas like a proper mathematician he could go as fast as thought. As it happens, Alan has become fascinated by the incarnations of pure ideas in the physical world. The underlying math of the universe is like the light streaming in through the window. Alan is not satisfied with merely knowing that it streams in. He blows smoke into the air to make the light visible. He sits in meadows gazing at pine cones and flowers, tracing the mathematical patterns in their structure, and he dreams about electron winds blowing over the glowing filaments and screens of radio tubes, and, in their surges and eddies, capturing something of what is going on in his own brain. Turing is neither a mortal nor a god. He is Antaeus. That he bridges the mathematical and physical worlds is his strength and his weakness.
What Stephenson describes is not just the inspired application of mathematics to physics and biology and wartime exigencies, intellectually beautiful though that is. When Turing operated at full extension, understanding and action were of a piece. He might not have been a Leonardo or Goethe, and he might have renounced the very idea of the human soul, but he was complete in his own fashion as few people, and only the most exceptional scientists, can ever hope to be.
For all his patent excellence, Turing struck others at Bletchley as disturbingly odd — even more so than the stereotypical mathematical eccentric. One secretary would express the common view with cruel bluntness: “People thought he was a bit of a weirdo.” He used a length of rope for his belt. He kept his treasured tea mug chained to the radiator in the work room. Bothered by hay fever, he bicycled to work from his billet in a nearby town wearing a gas mask. His bicycle’s chain habitually would disengage after a certain number of wheel revolutions, so rather than fix the bike, he counted the revolutions and reversed the pedals at the critical moment. He stammered, perhaps tactically, sputtering “Ah-ah-ah-ah-ah” as he struggled for a thought to achieve coherence. Under pressure his voice would squeak like a squeeze toy. He walked around looking intense, preoccupied, and gloomy — though not unexpectedly, given the burden of responsibility he was carrying.
Women — who were as abundant at Bletchley as they had been scarce at school and university — gave him the willies, and he would visibly shrink from the possibility of contact when most came near. Yet he fell in something like love, maybe, in a way, with the skillful cryptanalyst Joan Clarke, or at any rate admired her sufficiently to become engaged to her, briefly. He plainly esteemed her, and in particular thought well of her mind; he said he could talk to her as though she were a man. Although he told her of his “homosexual tendencies,” he did not exactly come clean about his homosexual practices. Hodges sums up the common attitude widespread at the time toward marriages of convenience: “Many people, in 1941, would not have thought it important that marriage did not correspond to his sexual desires; the idea that marriage should include a mutual sexual satisfaction was still a modern one, which had not yet replaced the older idea of marriage as a social duty.” But in the end Turing broke off the engagement, quoting to his not-quite-beloved the closing lines of Oscar Wilde’s The Ballad of Reading Gaol, written during Wilde’s incarceration for “gross indecency”:
Yet each man kills the thing he loves,
By each let this be heard, Some do it with a bitter look,
Some with a flattering word,
The coward does it with a kiss,
The brave man with a sword!
At this hybrid institution that Bletchley Park was, part civilian and part military, Turing’s blatant disregard for the spit-and-polish mandatory in the armed forces drove the brass hats to distraction. When he wanted to learn to shoot a rifle, he signed up for the Home Guard training course, and became quite an accomplished marksman. In the document he signed, he was asked if he understood that he was now subject to military discipline; Turing wrote no. No one noticed the clearly wrong answer at the time. Then when he happened to report to an Army officer and turned up with his usual slovenly civilian attire and slouching manner, the officer dressed him down for failure to observe military propriety. Turing replied that he had explicitly declared in writing that he was not to be bound by soldierly regulations. The exasperated officer, not knowing what else to do with somebody like Turing, dismissed him.
Fortunately for Turing and the Allies, Winston Churchill fully appreciated the importance of cryptanalysis, as he had from his days as First Lord of the Admiralty overseeing Room 40 during the First World War, and he saw to it that these brain workers were as respected as fighting men. When Churchill visited Bletchley Park to boost morale in September 1941, Turing was almost too shy to meet him. But that October Turing’s was the lead signature on a bold letter telling the prime minister that their pressing needs for more staff and equipment were being ignored by the chieftains; Churchill commanded that they get whatever they needed, and marked the order with his famous imperative, “ACTION THIS DAY.” Like so much else in the war, the achievement of Bletchley Park could not have been possible without the man in charge.
By the end of 1943, Bletchley Park was running with the efficiency of an industrial assembly line, and Turing’s singular mind was no longer required there. He sought and was granted a transfer to Hanslope Park, ten miles from Bletchley, a Secret Intelligence Service outfit so secret the staff “were working in a dream war themselves, one in which they knew neither the significance of what they were doing, nor what anyone else did,” as Hodges writes with obvious malicious delight. It was the perfect spot for Turing to initiate the work he was uniquely made for: “to build a brain,” as he stated his intention. The project would be the practical fulfillment of his guiding theoretical passions.
In June 1945, the National Physical Laboratory near London recruited him to work on developing a digital computer — just the thing to capture Turing’s attention. His work there would concentrate on what his boss called the Automatic Computing Engine. The set-up appeared highly promising, but Turing had a hard time getting the NPL to realize its promise. That his proposed engine would operate using binary arithmetic, so that “electronic switches could naturally represent ‘1’ and ‘0’ by ‘on’ and ‘off,’” as Hodges explains, impressed mostly by its strangeness those colleagues and superiors who would fund and construct the machine. “To an engineer, in particular, it would come as a revelation that the concept of number could be separated from its representation in decimal form…. He saw as obvious what to others was a jump into confusion and illegality.” His innovative use of programming instead of hardware was instrumental in the development of Baby, the first electronic stored-program computer.
To produce a machine that was a calculating marvel did not begin to circumscribe the philosophical scope of his project. He was exploring the similarity and difference — if there was a difference — in the function of machine brains and human ones. He boldly suggested that appearance was as good as actuality when it came to identifying the machine’s behavior: If it appeared to be thinking, then we may as well say it is thinking. The famous imitation game, also known as the Turing test, was designed to demonstrate whether a machine could appear to be a person.
There were two stages to the game. First Turing placed a man and a woman in a closed room with a teleprinter, and an interrogator in the next room. The interrogator would ask a series of questions in the attempt to identify which one was the man and which one the woman. The man would try to convince the interrogator that he was a woman by giving misleading answers, while the woman would try to help the interrogator by giving truthful answers. Then Turing replaced the man being questioned with a computer, in order to see if it could pass for human. Turing would write in his 1950 article “Computing Machinery and Intelligence” for the journal Mind, “We now ask the question, ‘What will happen when a machine takes the part of A [the man] in this game? Will the interrogator decide wrongly as often when the game is played like this as he does when the game is played between a man and a woman? These questions replace our original, ‘Can machines think?’” Turing expected that it would take fifty years before machines might fool humans.
With the war’s end, Turing’s motives in studying the machine mind “had more to do with the paradox of determinism and free will, than with the effecting of long calculations,” Hodges writes. Turing’s unusual theoretical model of the human brain was of paramount significance here. He had described his eponymous machine’s “states of mind.” Thus, if such a machine were actually to be built, it would be a virtual brain. Turing did not argue that the human brain is nothing but a machine, but rather held that mind and machine operated on the same principles of logic; he observed a distinction between reductionism and equivalence. Physics, chemistry, behaviorism — “which spoke of reducing psychology to physics” — were all beside the point. “The thesis was that ‘mind’ or psychology could properly be described in terms of Turing machines because they both lay on the same level of description of the world, that of discrete logical systems.”
The logical system of the Turing machine, however, embodied an absolute determinism that he was disinclined to attribute to the human mind or even to the universe described by classical physics, running in perfect comprehensible lockstep. Turing wrote:
It will seem that given the initial state of the machine and the input signals it is always possible to predict all future states. This is reminiscent of Laplace’s view that from the complete state of the universe at one moment of time, as described by the positions and velocities of all particles, it should be possible to predict all future states. The prediction which we are considering is, however, rather nearer to practicability than that considered by Laplace. The system of the “universe as a whole” is such that quite small errors in the initial conditions can have an overwhelming effect at a later time. The displacement of a single electron by a billionth of a centimetre at one moment might make the difference between a man being killed by an avalanche a year later, or escaping. It is an essential property of the mechanical systems which we have called “discrete state machines” that this phenomenon does not occur.
Turing’s argument is tellingly confused here. Laplace was imagining a perfect mind that did not make any errors of observation whatsoever even on the order of the infinitesimal. Perhaps Laplace was imagining the ideal physicist taking stock of the sum of material knowledge, but the only mind that could possibly encompass Laplace’s universe is neither human nor mechanical but divine, as that is traditionally imagined by men of faith rather than science. The question of the practicability of human intelligence does not figure at all in this scientific fantasy.
The structure of the Turing machine is just like that of the perfect Laplacian mind. He is describing a machine whose mind operates with a perfection that the human mind will never possess — yet that is also constrained by necessity as the human mind is not. In Laplace’s universe, the human mind must be logically structured exactly as the divine mind is; and the human mind must also be logically structured exactly as the machine mind is. Thus the divine mind is bound by the same necessity that binds the machine. Nothing is permitted free will, except perhaps the Creator until he fixed the laws of matter. The universe is a machine that cannot run any differently from the way it does.
Turing, then, is making room for human error in a universe conceived to allow no free will. Determinism is possible only if the human mind, bound by Newtonian laws of motion to inalterable cosmic destiny, is incapable of choosing any differently. But the human propensity for error, which he rightly highlights, implies unlimited contingency, an endless branching of uncertainties. Should the Turing machine be programmed with all the information about every particle in the universe it still could not definitively plot the cosmic future: The wayward minds and actions of human beings would vitiate the mathematical formulas.
This is the long way around to settling the question of free will and determinism as Samuel Johnson did, when his pious mind was under assault from the Enlightenment powers of philosophical darkness: “We know our will is free, and there’s an end on’t.” The mind of man and that of the Turing machine are essentially different in kind — not just in the material conditions of their existence but in the way they function, their logical structures. Human minds cannot be described as Turing machines, no matter what the experts say. When Turing speaks of the “essential property” of the “discrete state machines” — that they operate entirely predictably and without functional error within their ambit — he implies that there are no accidents, no unforeseeable contingencies, in the thought-world of the mechanical brain. But human reality is full of them.
The story of Turing’s downfall hardly fits with what had gone before in his life: The heroic fate of his extraordinary mind is reduced to the sad plight of the common lot. On the cruising ground of Manchester’s Oxford Street in December 1951, Turing picked up a nineteen-year-old man, Arnold Murray, unemployed, down-at-heel, and with a conviction for petty theft on his rap sheet. For both men, Hodges writes, this encounter was redeemed from the usual coarseness by their desire for something more than instant anonymous sex. “Fair and with blue eyes, undernourished and with his thin hair already receding, desperate for better things and more receptive than so many educated people, Arnold touched Alan’s soft spot for lost lambs, as well as other chords…. By making invitations to lunch and to his home, Alan had already offered a good deal more than would usually be expected of a street encounter.”
They would see each other a few times over the next six weeks or so; Murray spent the night at Turing’s house more than once, giving the rendezvous the respectability of an “affair” in Turing’s eyes. He had offered Murray money after their first night together, but Murray had his dignity, and would not stoop to be a “renter.” However, Turing found a few pounds missing from his wallet after that night. Murray hotly denied the theft; Turing did not quite believe him, and wrote to break off their acquaintance. But when Murray showed up again anyhow, Turing gave him another three pounds, and then seven pounds more the next time.
When Turing’s house was robbed, he thought Murray the robber. Murray claimed he did not do it, but said he knew who likely did. He had told an acquaintance, Harry, about Turing, and Harry had tried to enlist him in the burglary of Turing’s place, but he had refused. So Turing told the police about Harry, and Harry told the police that Murray, who had “business” at Turing’s house, had put him on to his victim. When Turing tried to conceal Murray’s identity from the police, who already knew about him, Turing was caught in a lie, and in his consternation proceeded to tell the detectives all about his “affair.” He wrote them a five-page statement, replete with explicit detail. Hodges writes, “Relieved of the usual necessity to translate human life into police language, they were most appreciative of what was ‘a lovely statement,’ written in ‘a flowing style, almost like prose,’ although ‘beyond them in some of its phraseology.’ They were particularly struck by his absence of shame. ‘He was a real convert…. He really believed he was doing the right thing.’” The prosecutor was similarly appreciative, and secured convictions against Turing for gross indecency several times over.
David Leavitt gives a brusque summation of the sudden and irreversible change of course that Turing’s life underwent.
The little that was left of Alan Turing’s life after his arrest was a slow, sad descent into grief and madness. Tried on morals charges, he was “sentenced” — in lieu of prison — to undergo a course of estrogen treatments intended to “cure” him of his homosexuality. The estrogen injections had the effect of chemical castration. Worse, there were humiliating side effects. The lean runner got fat. He grew breasts. Through it all he continued to work, soldiering on with the resilience he had had to learn at Bletchley.
But his resilience had its limits: Leavitt, like Hodges, believes but cannot be certain that Turing fell into a sepulchral depression and killed himself by eating a few bites of an apple dusted with potassium cyanide on June 7, 1954. The coroner’s inquest ruled his death a suicide by poison, “while the balance of his mind was disturbed.”
Leavitt dismisses the “sort of conspiracy” on the part of Turing’s mother and his friends “to propagate the myth that his death was the result not of suicide but of a scientific experiment gone awry.” Hopeful that her beloved son’s immortal soul might find salvation, Sara Turing wrote in her 1959 memoir, “Many friends, either by reason of his temperament and recent good spirits, or because of ‘his unlimited flow of ideas and great enthusiasm for putting them into practice,’ have been led to believe that his death was caused by some unaccountable misadventure. Besides, his inadvertence alone had always involved the risk of an accident.” Their thinking was that Turing kept cyanide in the house for chemical experiments, and he was habitually so careless that he might have inhaled cyanide gas from an experiment he had cooking or dipped his fingers in the stuff and accidentally transferred the poison to the apple.
Leavitt and Copeland also broach the possibility that Turing was murdered by government agents who were silencing a dangerous security risk and who staged the apparent suicide. The treasonous Cambridge spies Guy Burgess and Donald Maclean had defected to the Soviet Union in 1951, and they were doubly notorious because of their homosexuality. So it was not implausible for the authorities to think that Turing would make a likely prospect for turning by the KGB, and he had indeed been under close surveillance after his arrest. Traveling abroad for safe sex once the hormone injections had worn off, he had befriended a Norwegian man and invited him to come visit in Manchester; but when the friend tried to enter the U.K., the authorities, apparently monitoring Turing and his would-be visitors, abruptly sent him back home. One wonders how far they would have been willing to go to ensure Turing’s continued good behavior. However, it actually seems more likely that such unwanted oversight would have driven Turing to desperation, rather than indicate the Secret Intelligence Service’s willingness to kill him. Suicide does appear the most likely cause of death.
It is difficult, under the current dispensation, when celebratory rainbows have bedecked the White House, to imagine the disgust and horror that so many otherwise decent people in Turing’s day felt and expressed at the presence of gay people in their midst. Turing himself might well have seen his sexuality as wrapped unnecessarily in the enigma of determinism versus free will, with nature taking one side and society the other, when there should have been no conflict at all. It had long appeared clear to his mind that nature had made him the sort of sexual being he was, and he saw no reason to wish himself otherwise, but his behavior was treated as a matter of wrong moral choice by the guardians of the proprieties, who exercised their dominion over his very sexual parts. Theoretical reason had it all over unreason, as usual; yet unreason prevailed in practice, condemned him to a period of enforced misery, and perhaps provoked him to choose death over an oppressive hole-and-corner life — or even caused him to think, as suicides often do, that he had no choice but to die.
It is not fanciful to suggest that Turing conceived the human mind as cognate with determined mechanism partly in response to his personal dilemma as a man who was not permitted by society to be what he felt that he was by nature. To follow reason and nature, he discovered, will literally cost you your manhood at the hands of your unreasoning fellow men; and to add insult to injury, science will do its worst to abet the oppressor. It must have been hard to view his own scientific subject with pure disinterestedness when he had so vital a stake in his findings. In so far as he was a student of the mind, he was of necessity his own subject. In a letter to a friend telling of his arrest, he composed a wry and wincing pseudo-syllogism that linked his sexual criminality to his most cherished scientific work: “Turing believes machines think / Turing lies with men / Therefore machines do not think.” At least in the public mind, who he was threatened to discredit what he thought. The sensation of unfreedom that bedeviled the most intimate aspects of his life could not but have shaped his character, and one can readily imagine its impinging upon his vocation as a thinker.
But then one might simply be imagining such a thing; this speculation may be completely off the mark. Turing might well have been entirely capable of separating his singular mathematical mind from the bodily and psychic concerns that all people have in common but that were peculiarly contorted by his official status as a gay man subject to legal persecution. His gift for pure abstraction was remarkable enough that it just might have operated free of such all-too-human cares. Yet what he endured, and in the end could no longer endure, cannot but figure in the picture as one considers what his life meant. Turing’s was perhaps the most tragic of eminent scientific lives. That he should have suffered and died as he did, at the age of forty-one, leaves one choking with rage and shame. But it is for the splendor of his intellect that he must be remembered. Too few people do remember. He is famous enough, mostly thanks to his wartime work, that mediocre plays and downright bad movies are made about him; but his name does not resound like that of Darwin or Einstein. Billions of people who use their laptops or phones as though they were natural human appendages have never heard of him, who presided at the beginning. That is our loss. We ought to honor as one of the great heroes of modernity this strange and extraordinary man who brought mathematics down from the heavens to serve the lives of ordinary human beings.
During Covid, The New Atlantis has offered an independent alternative. In this unsettled moment, we need your help to continue.